When choosing any kind of sensor, it is important to answer the following questions:
- What are we measuring and under which conditions?
- What are the relevant factors concerning our measurements?
- What do we wish to gain from our measurements in terms of quality, quantity, and price?
What follows is a short summary of the characteristics.
Ground isolation
Accelerometers with ground isolation usually have an isolated mounting base and an isolated mounting screw, or in some cases, the entire accelerometer case is the ground isolated.
Ground isolation becomes important when the test articles surface is conductive and at ground potential. A difference in ground voltage levels between the electronic instrumentation and the accelerometer may cause the ground loop resulting in erroneous data.
Sensitivity
The sensitivity is the first characteristic normally considered. Ideally, we would like a high output level, but here we have to compromise because high sensitivity normally requires a relatively big piezoelectric assembly and consequently a relatively large, heavy unit. In normal circumstances, the sensitivity is not a critical problem as modern preamplifiers are designed to accept these low-level signals.
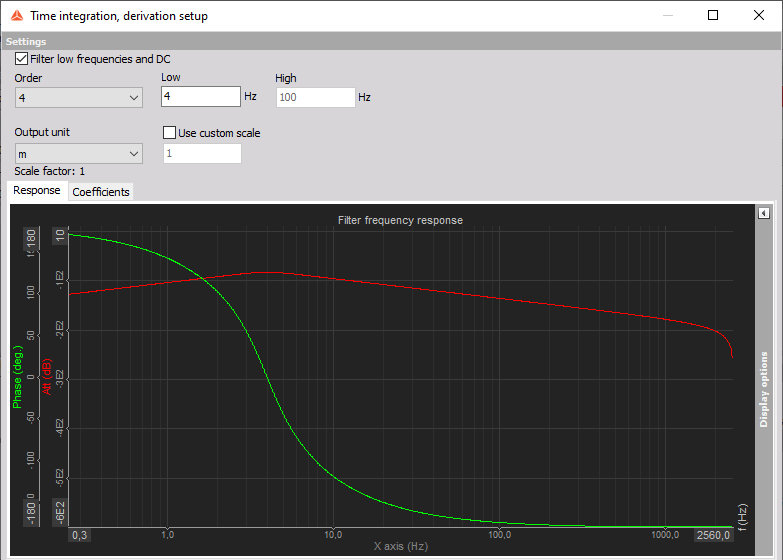
Low-frequency range
The requirement for vibration measurements is usually that the sensor has a lower high pass cutoff than the frequencies of interest of the devices currently being tested. On a rotating machine normally running with 50 Hz, we can choose a sensor with a 5 Hz cut off. When measuring building or ship vibration, this level must be very low. Another important thing, to consider, is bandwidth since the lower it gets, the longer is recovery times from shocks or overloads. Also, the amplifier should follow the bandwidth of the sensor. It is nice if the amplifier has at least two ranges in order to be more flexible in measurements. A typical application for low-frequency measurements is the paper mill rolls. They have a frequency of 1-5 Hz, where the user would need a sensor with 0.3 Hz or less bandwidth. For those applications, charge or IEPE are most suitable. If we need to measure the static acceleration then a different sensor technology, like MEMS sensors, is needed.
The low-frequency range, over which the accelerometer gives a true output, is limited at the low-frequency end in practice, by two factors. The first is the low-frequency cut-off of the amplifier which follows it. This is normally not a problem as the limit is usually well below one Hz. The second is the effect of the ambient temperature fluctuations, to which the accelerometer is sensitive. With modern shear-type accelerometers, this effect is minimal, allowing measurements below 1 Hz for normal environments.
Bandwidth (frequency range)
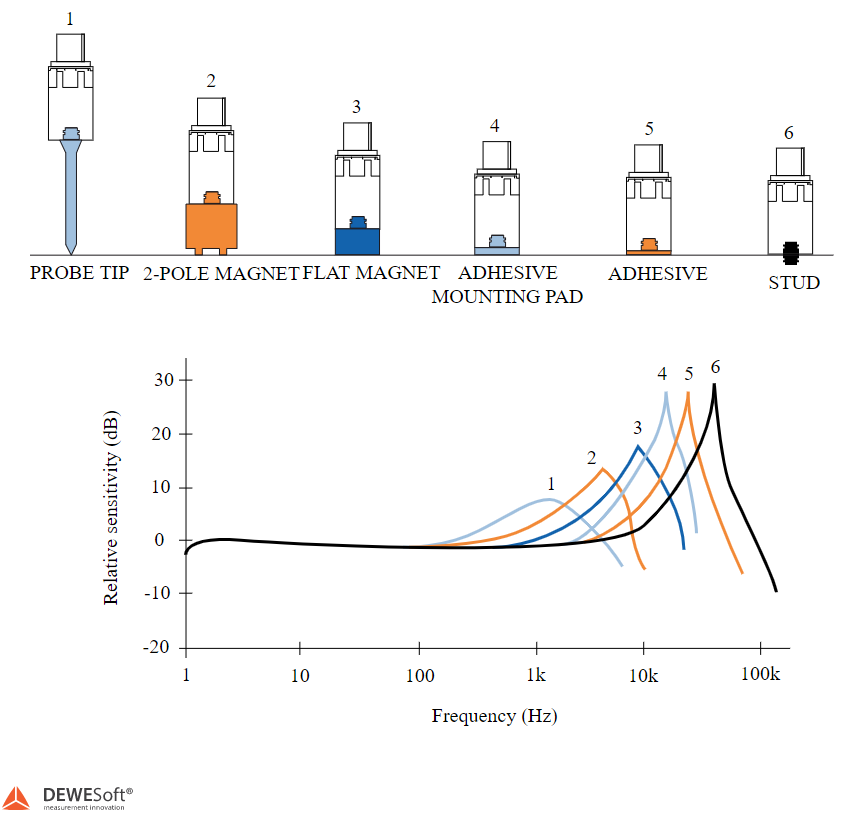
Mechanical systems tend to have much of their vibration energy contained in the relatively narrow frequency range between 10 Hz to 1000 Hz but measurements are often made up to say 10 kHz because interesting vibration components are often present at these higher frequencies. Therefore, we must ensure, when selecting an accelerometer, that the frequency range covers the range of interest. The upper limit is determined by the resonant frequency of the mass-spring system of the accelerometer itself. As a rule of thumb, if we set the upper-frequency limit to one-third of the accelerometer's resonance frequency, we know that the vibration component measured at the upper-frequency limit will be in error by no more than + 12%.
With small accelerometers where the mass is small, the resonant frequency can be as high as 180kHz, but for the somewhat larger, higher output, general-purpose accelerometers, resonant frequencies of 20 to 30kHz are typical.
We need to be careful about the increased sensitivity at sensor high-frequency end due to its resonance. Reading in this area will be too high but can be removed in the frequency domain if sensor transfer characteristics are known (by using transfer curves in Dewesoft).
Amplitude range
Charge sensors have the biggest amplitude ranges (specially designed shock sensors can have more than 100 000 g amplitude range), but IEPE is also fairly high (up to 1000 g). MEMS sensors usually have a very limited range (up to a few hundred g). For general purposes, it is best to use IEPE, whereas for high levels piezoelectric sensors are better. Sometimes (for example for seismic applications) an accelerometer with high sensitivity is required (2 g or lower range).
Maximum shock level
The charge sensors are the least sensitive to shock. They can sustain up to 100 000 g of shock while IEPE can usually take not more than 5 000 to 10 000 g. MEMS sensors are even more sensitive to shock.
Noise level
The residual noise level defines the lowest amplitude level of what the sensor will measure. This is also the reason why we should take a sensor with the optimum measurement range because sensors with a higher range will also have a higher noise level.
IEPE sensors have a very high dynamic range (we can see signals better than 160 dB below the maximum range). Charge sensors are similar, but we need to consider that the noise can be easily generated in the cable. MEMS sensor is much worse in a dynamic range limited by internal electronics.
Temperature range
All the sensors, that include electronics, have a limited high-temperature range, up to 130 deg C. The temperature range of charge sensors is much higher - even up to 500 deg C. Please note however that this also requires a high-temperature cable.
All piezoelectric materials are temperature dependent so that any change in the ambient temperature will result in a change in the sensitivity of the accelerometer. Piezoelectric accelerometers also exhibit a varying output when subjected to small temperature fluctuations, called temperature transients, in the measuring environment. This is normally only a problem when very low level or low-frequency vibrations are being measured. Modern shear-type accelerometers have a very low sensitivity to temperature transients. When accelerometers are to be fixed to surfaces at higher temperatures than 250°C, a heat sink and mica washer can be inserted between the base and the measuring surface. With surface temperatures of 350 to 400°C, the accelerometer base can be held below 250°C by this method. A stream of cooling the air can provide additional assistance.
MEMS sensor temperature range is limited by internal electronics (from -40°C to 125°C).
Weight
In some applications, like modal testing, weight can be a big factor due to the mass loading effect. The added mass to the structure changes the dynamic behavior, so ideally a sensor should have no mass at all.
That is kind of hard to achieve by normal design, but we can use laser contactless sensors in such cases. As a general rule, the accelerometer mass should be no more than one-tenth of the dynamic mass of the vibrating part onto which it is mounted.
Ground loops
The ground loop currents can flow in the shield of accelerometer cables because the accelerometer and measuring equipment is earthed separately. The ground loop is broken by using an isolated sensor, an isolated amplifier or electrically isolating the accelerometer base from the mounting surface by means of an isolating stud.
Cable noise
Cable noise is mainly the issue of piezoelectric accelerometers having a high output impedance. These disturbances can result from triboelectric noise or electromagnetic noise.
Triboelectric noise is often induced into the accelerometer cable by the mechanical motion of the cable itself. It originates from local capacity and charge changes due to dynamic bending, compression, and tension of the layers making up the cable. This problem is avoided by using a proper graphitized accelerometer cable and taping or gluing it down as close to the accelerometer as possible.
Electromagnetic noise is often induced in the accelerometer cable when it is placed in the vicinity of running machinery.
Transverse vibrations
Piezoelectric accelerometers are sensitive to vibrations acting in directions other than coinciding with their main axis. In the transverse plane, perpendicular to the main axis, the sensitivity is less than 3 to 4% of the main axis sensitivity (typically < 1%). As the transverse resonant frequency normally lies at about 1/3 of the main axis resonant frequency this should be considered where high levels of transverse vibration are present.
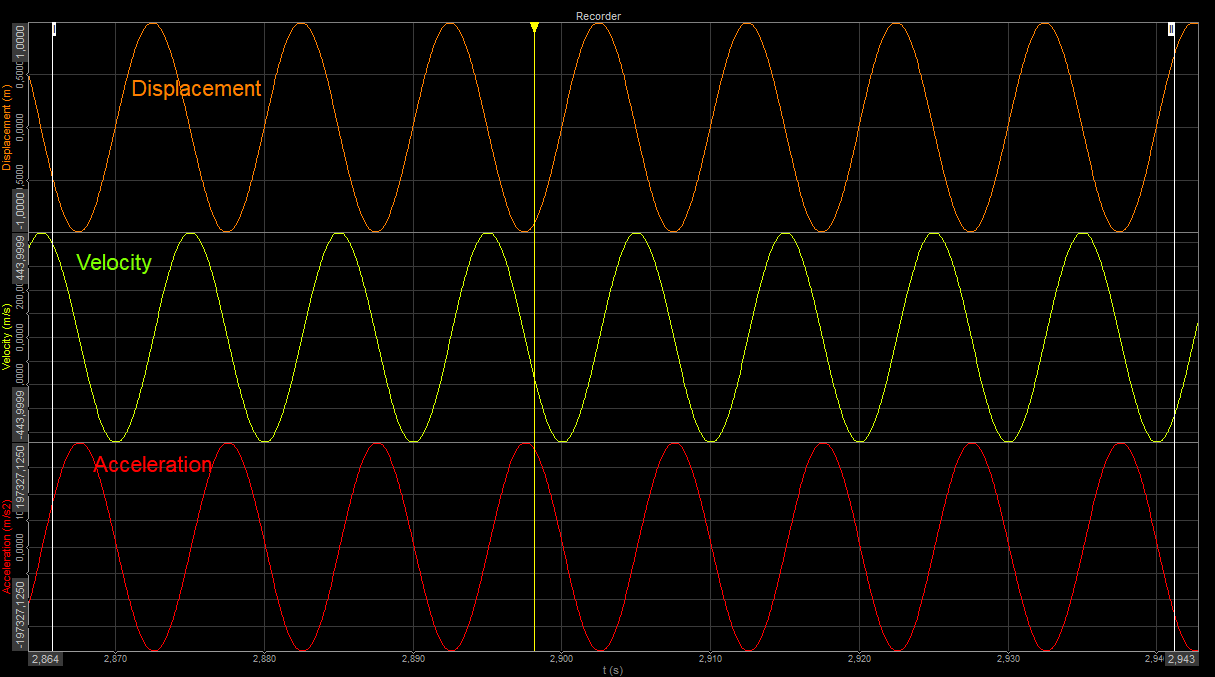
 Image 1: Vibrations caused by an unbalanced wheel
Image 1: Vibrations caused by an unbalanced wheel Image 2: Vibrations caused by a drilling machine
Image 2: Vibrations caused by a drilling machine